A compact gearbox

This post is about mechanical engineering, which is not really one of my specialities. The problem I set myself was to make a compact gearbox with a high ratio and low friction. This would be useful, for example, to connect a large, slow wind turbine to a fast, small generator, or to drive a large, slow bicycle wheel using a fast, small motor.

This is an idea I came up with up years ago, but never published until now.

Why are gearboxes big?

If you take a design for a gearbox, scale it down to the size of a matchbox, and build it, then it will work, but if you put any power through it then it will break. The gear teeth will shear off, or the gears will buckle and bend. If you make the gearbox huge, then it will transmit a lot of power, but it will be heavy, expensive, and in the way. A good design will be a compromise between these conflicting requirements.

The problem is harder still if you want a gearbox with a high ratio. The naive approach is to have one very big gear meshing with one very small gear. However, because the small gear has very few teeth, all the force will be transmitted through just one or two teeth. The teeth will get hot and wear out, especially on the small gear, which therefore cannot be made as small as you would like. And that forces the big gear to be enormous.

Another way to make a high-ratio gearbox is to chain together many moderate-ratio gearboxes. However, that too makes the gearbox large, heavy and expensive. It also makes it inefficient, because the losses in each gearbox compound.

My design

My design is based on planetary gears. The small, fast-rotating "sun" gear is green (10 teeth), and the large, slow-rotating "ring" gear is blue (40 teeth). The red stator has 50 teeth. The light and dark grey planetary gears (20 and 10 teeth respectively) orbit the centre.

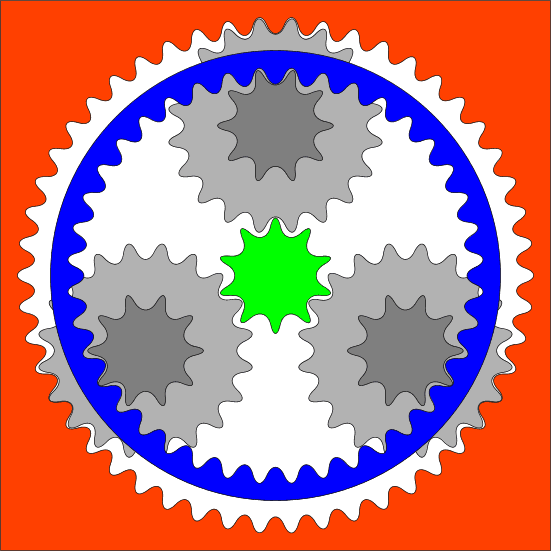
16-to-1 gearbox

This gearbox fits in the space of a single 50-tooth gear: about the same as a 40-tooth gear meshing with a 10-tooth gear. As shown it has a 16:1 ratio, while the simpler design achieves only 4:1.

My design is stronger. The sun gear meshes with three other gears, rather than just one, and can therefore transmit three times the power of the simpler design. The large forces on the blue ring and the red stator are distributed over many teeth.

In my design the green and blue gears are coaxial. This in principle eliminates all the forces on the bearings that hold the gears in place (not shown in the diagram), and makes the gearbox more efficient. In my design energy is only lost where the gear teeth rub against each other.

Calculating the ratio

Lets use a unit of distance equal to one tooth spacing. Imagine the green sun gear makes δ revolutions. We will work out the number of revolutions made by the blue ring gear. You can imagine δ to be tiny if you find it easier, but the calculation will work even if δ is large.

Imagine a straight lever from the top of the green sun gear to the top of the red stator. The lever is a diameter of one of the planetary gears; you could imagine drawing it on with a pencil.

The bottom of the lever moves a distance 10δ, because the sun gear has 10 teeth. The top of the lever does not move, because it meshes with the stator. The centre of the lever therefore moves a distance 10δ / 2: the average of 10δ and 0.

The dark grey gear has half as many teeth as the light grey gear. Therefore, the top of the dark grey gear is half way from the centre of the lever to the top. Therefore, when the centre moves 10δ / 2, the top of the dark grey gear moves 10δ / 4.

The top of the dark grey gear meshes with the top of the blue ring, which therefore also moves 10δ / 4. The blue ring has 40 teeth, so the number of revolutions it makes is (10δ / 4) / 40, or δ / 16.

In summary, the lever (the planetary gear) gives a mechanical advantage of 4:1, and the larger number of teeth on the blue ring gear compared to the green sun gear gives another 4:1 advantage, for an overall ratio of 16:1.

Increasing the ratio

The main benefit of my design, however, is that it is easy to increase the ratio of the gearbox:

This increases the ratio dramatically, without making the gearbox any bigger.

Let's calculate the ratio. The first part is the same. The centre of the lever still moves a distance 10δ / 2. However, the top of the dark grey gear is now 95% of the way from the centre of the lever to the top (19 / 20). Therefore, when the centre moves 10δ / 2 the top of the dark grey gear moves just 10δ / 40. The blue ring gear also moves by that amount, so the number of revolutions is makes is (10δ / 40) / 49, or δ / 196.

In summary, the lever gives a mechanical advantage of 40:1, and the ratio of the blue to the green gears gives another advantage of 49:10, for an overall ratio of 196:1.

Limitations of the design

Perhaps counter-intuitively, the failure mode of my high-ratio design (when it is overloaded) will be to shear off the teeth on the blue or red gears, since these bear much greater forces than the puny green gear. The design mitigates this failure mode in several ways:

Shrinking the sun gear, say to just 5 teeth, would not make the gearbox weaker, and would increase its ratio still further. However, shrinking it too much will result in the planetary gears interfering with one another. Conversely, enlarging the sun gear would allow more planetary gears to fit, strengthening the gearbox at the expense of reducing its ratio a bit.

Fundamentally, the limit on the torque (and therefore power) that the gearbox can transmit is the force that can be applied to a tooth of the blue gear, multiplied by its radius, by the number of teeth that mesh with each planetary gear, and by the number of planetary gears. The radius of the blue gear is already as large as will fit in the gearbox. Making the teeth smaller increases the number that mesh, but weakens them by about the same amount, resulting in no advantage. Increasing the number of planetary gears reduces their size, and therefore the number of teeth of each that mesh with the blue ring, resulting in no net advantage.

Therefore, for a given material and size of gearbox, my design is theoretically quite difficult to beat. If you don't mind its other disadvantages, something like a cycloidal drive might manage.

Last updated 2025/05/16